1. A triangle has integral sides. Its perimeter is 2006. One of the sides is sixteen times another side. The number of such triangles is [ ]

A) 0 B) 1 C) 2 D) 3 E) 4

2. Given that ABC is an arbitrary triangle. The number of points P in the interior of the triangle such that APB = BPC = CPA is [ ]

A) 0 B) 1 C) 2

D) not necessarily 0, not necessarily 1, but either 0 or 1

E) not necessarily 1, not necessarily 2, but either 1 or 2

3. The number of rhombi that can be inscribed in a parallelogram such that each side of the parallelogram contains precisely one vertex of the rhombus is [ ]

A) 0 B) 1 C) 0 or 1 D) 0 or 2

E) infinitely many

4. In this question a polynomial means a polynomial with real coefficients. P(x) is a polynomial of degree 2006 and P(x) 0 for all real x. Then [ ]

A) P(x) must have at least one complex root

B) P(x) cannot have 2006 distinct real roots

C) P(x) is a product of 1003 quadratics, none of which has linear factors.

D) P(x) cannot have a polynomial factor of the form (x-k)²⁰⁰⁵

E) none of these

5. If 0<axb, 0<ayb and 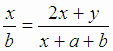, then [ ]

A) a=x=y=b B) a=x<y=b C) a=y<x=b D) a=yx=b

E) none of these

6. p, q, r are given real numbers. Consider the polynomials (q-r)(x-q)(x-r) + (r-p)(x-r)(x-p) + +(p-q)(x-p)(x-q) + k for all real k. Then [ ]

A) there exists a k such that the degree of the polynomial is 2

B) there exists a k such that the degree of the polynomial is 1

C) there exists no k such that the degree of the polynomial is 0

D) there exists no k such that the degree of the polynomial is undefined

E) none of these

1. A lattice point is a point both of whose coordinates are integers. A is (0, 0), B is (4012, 6018). If n is the number of lattice points lying on , then n = _________

2. Two circles of equal radius r are inscribed in a semicircular sector of radius ‘1’ such that they touch each other externally. Then r = ___________

3. In a triangle ABC, D, E are points on respectively such that and DE = 5. If AB = 8 and bisects , then the length of = ____________

4. 1 + = ____________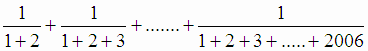

5. The characteristic of log₃ 2006 is __________

6. If the positive integer m is such that 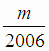is closest to , then m = ________

1. A(0, 0), B(4012, 0), C(4012, 6018), D(0, 6018) determine a rectangle which is the union of 4012 x 6018 unit squares. Determine the number of these unit squares which have a nonempty intersection with .

2. P is a point not lying on a line l. Explain how you construct an equilateral triangle PQR such that l.

3. Let E be the midpoint of the side of ABC and let F be chosen in so that AC=3FC. Determine the ratio of the areas, ABEF : EFC.

4. Does the equation 2006 = (x+y)² + 3x + y have a solution in nonnegative integers?

5. Without removing brackets at any stage factorize 2y(y+z)-(x+y)(x+z)

6. Factorize the polynomial in x: (t²+1)x³ – (t²-1)x² – (t²+1)x + (t²-1)

1. Treating the equalities log₁₀2 = 0.3010 and log₁₀3 = 0.4771 as exact, prove that the sum to 30 terms of the series 2 + 6+ 18 + 54 + ….. exceeds 2x10¹⁴.

2. Factorize a⁵(b-c) + b⁵(c-a) + c⁵(a-b)

3. Prove that (n+1) is either itself a prime or a product of primes for every positive integer n.

4. A triangle ABC is turned about the vertex A in the plane of the triangle into the position . If bisects , prove that bisects .

5. P is a point in the plane of parallel lines l, m. Explain how you locate a point Q on l and a point R on m such that and are equal in length and perpendicular to each other. Discuss only the case when P lies between l and m.

6. A, B, C are respectively (1002, 1005), (2004, 2001) and (1003, 1000) and P₀ is (1, 4). Points P_i, iN are such that

A is the midpoint of

B is the midpoint of

C is the midpoint of

Estimate the ratio of lengths 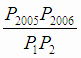.